What a Shock - Newton Correct!
By Lou Trunk – Professional lane installer
Two time winner of BPAA Special Projects Award
USBC National Tournament Lane Installer and/or Stand-By Service Manager since 1987
Over the past 24 months, along with the staff at Kegel, we have stepped up the topography testing of years prior by performing revolutionary experiments and gathering data from all over the world. We have been closely studying different lane shapes, creating formulas, having late night jams sessions, and watching 1000’s of bowling balls go down the lane trying to prove, and disprove, how topography affects the motion and the direction of the bowling ball as it rolls from foul line to off the end of the pin deck. Notice we didn’t write head pin. As you read the full series, you’ll come to understand why.
This series of articles may be the most important subject players, proprietors, tournament organizers and administrators of the game have ever read regarding the technical side of the modern day sport of bowling.
Newton Correct!
The “thought experiments” we, along with a very few others, have been executing in our minds for over 20 years, finally took to the lanes early November 2009 in the form of actual measured real life situations of lane topography, on which actual real life bowlers of various styles threw shots, which produced observable and CATS™ measured ball reactions.
The initial tests were exciting and invigorating to John Davis, Bill Mongeau, Ted Thompson and me, but probably not so shocking to Sir Isaac. Indeed, it appears that Newton’s First and Second Laws in fact apply to the game of Bowling.
In layman’s terms, these experiments involve three basics:
1. Momentum, (and the law of conservation of momentum): a body’s momentum equals it’s mass times it’s velocity p=mv (p is the symbol for Momentum).
2. Newton’s First Law of Motion, which states in the absence of force, a moving body will move in a straight line at constant speed.
3. Newton’s Second Law of Motion, which states when a force is applied to a body,acceleration will result in the direction of the force.
Most important with regard to Newton’s Second Law for our experiments, is that the net force on an object is equal to the time rate of change of its linear momentum.
For example, the more momentum a ball has, the more force will be needed to act upon the ball, in order to change the ball’s path by a certain distance.
In bowling, the gravitational force on a bowling ball comes from a lane’s tilts, depressions and crowns. And mind you, there is not a perfectly flat lane anywhere on this planet.
Most everyone in the bowling industry considers the lane surface as a two dimensional surface. A flat plane, or an X and Y axis, with the X axis being the width of a lane, and the Y axis being the length of a lane. If the lane was merely two dimensional, gravity would simply be a constant throughout any bowling ball’s journey down any lane. That is simply never the case, and the often unconsidered Z axis – the change in elevation – has a significant amount of influence on ball motion.
For our experiments we considered the force, momentum and inertia situations. The constants on repeated shots were mass (ball weight), lane surface, gravity, oil type and oil pattern; which combine to produce a certain ball path shape for a certain bowler with a certain ball on a flat surface. Then we changed only the topography, and that’s where the “shock” began. And it was shocking to us, but not to Sir Isaac Newton.
Slope per Board is the Key!
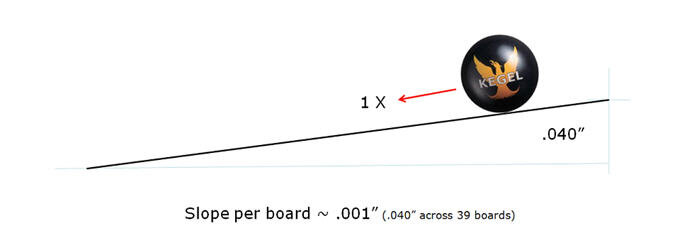
The first thing we must explain is the creation of a brand new term in bowling called, Slope per Board. With the invention of the Kegel Lane Mapper, by taking crown and depression readings of each and every board across the lane, and then adding the single crosstilt reading to each board, we can calculate the slope of each board at any distance on the bowling lane.
To fully understand the significance of this reading, we must understand that as the bowling ball travels down the lane from foul line to pin deck, it simply reacts to whatever gravitational force is acting on the ball on whatever specific board it is on at any one moment in time, and it doesn’t care about the slope of surrounding boards.
For instance, we know a bowling lane consists of 39 boards, and if a bowling lane is tilted high right 40/1000” (1 mm), which is the maximum allowable amount under the specification rules, that would give us a slope per board value of about 1/1000” (.025 mm) for each board on the lane.
If we double that crosstilt to be 80/1000” (2 mm), which is two times the allowable amount under the specification rules, that would give us a slope per board value of 2/1000” (.050 mm) for each board.
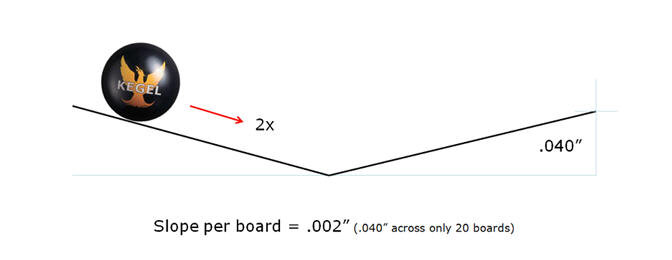
Another instance that would give us that same 2/1000" slope per board value, but be within current specification, would be a 40/1000” v-shaped crown or depression directly to the center of the lane (.040” slope/20 boards = .002” slope/board.)
The ball doesn’t care about the specification. It feels the exact same gravitational influence of .002” under each scenario – one scenario twice the allowable amount, and one perfectly within specification.
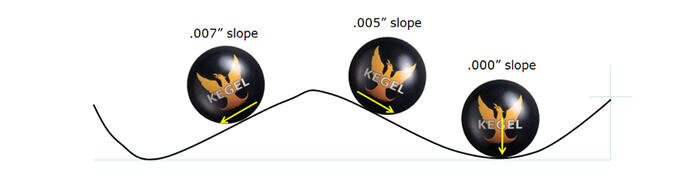
Further, as soon as we introduce crowns and depressions into the equation, that crosstilt slope per board value can increase significantly, or even decrease, and depending on which way the gravitational slope is, it will influence the bowling ball to the left or to the right as it travels down and across the lane surface.
What did we do?
We, so far had introduced a “force” to the ball, a Gravitational Force. We shaped a few of the adjustable Kegel Training Center lanes with consistent gravitational shapes relative to the lane, yet contradicting gravitational forces relative to the ball’s inertial path.
On one pair of lanes, we created as near a non-imbalanced gravitational force as we could, as flat as possible. This gives us a benchmark ball motion reaction where there is constant gravitational force on the bowling ball as it rolls down the lane.
On another pair, we created two opposite shapes.
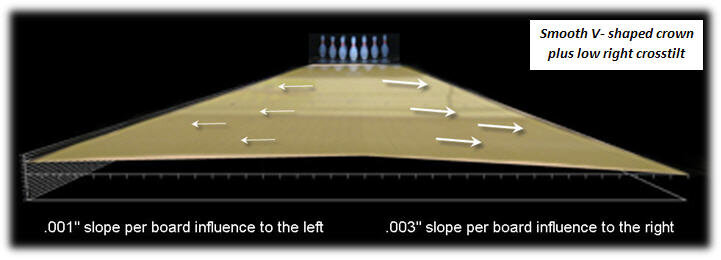
One lane had a legal gravitational imbalance of approximately .003” slope per board (SPB) low left for a right-hander playing anywhere from 1-20 board. We did this by creating a .040” low left crosstilt (.001”SPB), plus a .040” smooth V-shaped depression from both 1 boards to the 20 board (.002” SPB) which gives us that .003” per board slope effect toward the center of the lane for a right-handed player.
On this lane’s mate, we created the low right equivalent. We did this by reversing what we did on the companion lane.
It is important to note that this very shape yields only a .001” slope for a left-hander playing anywhere from boards 1-20 on his side, since the combination of the tilt and the crown/depression compound the slope for the right-hander but are partially counterbalancing for the left-hander.
And finally, just like the pictures above, we created two lanes with real world situations of a net gravitational imbalance of approximately .005” slope per board. One lane with a gravitational force towards the center of the lane, and the other gravitational force towards the right gutter for a right-handed player, which was again opposite but nearly flat for the left-hander because of the counter-balancing combination of the crosstilt plus the crown and depression.
What did we see?
Newton would be proud. The left-handers had all pairs about the same. The right-handers certainly did not. The relative effect on the bowling ball was proportional in three ways. First, there was nearly double the effect on a ball’s path at .005” slope per board as there was at .003” slope per board in the direction of the slope.
Secondly, the effect was proportionally less for higher ball speeds and greater for slower ball speeds. The faster the ball was thrown, the less boards the ball missed the intended breakpoint because of the gravitational effects of the lane topography.
Remember that the displacement caused by a gravitational influence is a function of the time spent on the influence, so it stands to reason: faster speed = less time on the influence = less displacement.
Sure enough, the differences in the two opposite gravity force lanes were proportionally greater for slower ball speed players. And third, lighter weight balls were proportionally more effected by a certain slope.
“Displacement caused by a gravitational influence is a function of the time spent on the influence.”
So at this point, what we had tested so far, were bowling lanes with a consistent gravitational force, either inward or outward, and bowlers of various speeds and ball weights relative to themselves – comparing a bowler’s data to his own data on the various shapes. Then we gathered data comparing bowlers to other bowlers. Bowler A playing straight up the 5 board and Bowler B playing 20 to a break point of 5.
For Bowler A, where the ball hit the pins was greatly different since his ball’s translation was almost continuously at a 90 degree angle to the gravitational force vector. The net change in impact position was greatest with this style on these opposite lane shapes.
Bowler B’s net change in impact position was not as significantly different as Bowler A’s, because Bowler B had the gravitational force displacing his ball at a slightly more obtuse angle (an angle greater than 90° and less than 180°).
The results for the two launch angles are very different and very significant.
It would appear that the nightmare pair for the down-and-in type player is one lane tilted left all the way and one lane tilted right all the way, because his ball is continuously influenced near perpendicular to his ball’s path throughout its travel from foul line to pins, so the impact point change is huge. As much as hitting the pocket on one lane and hitting only the 3 off the right (6-9-10 pins) on the other.
The boomer’s ball (Bowler B) had less perpendicular gravitational effects on its way down the lane both to and from the breakpoint in this all left slope or all right slope situation. The impact point doesn’t change as much as Bowler A, but the hitting power and shape of the ball path does.
Bowler B’s ball path shape was more of a curve on the all left slope covering far less boards. It was easier to control the shot, and it was less speed sensitive, but incurred a lower percentage pocket carry. On the all right slope Bowler B’s ball path shape was more of a skid-snap type reaction covering more boards but with less control. The ball was also more speed sensitive however it had a higher pocket carry percentage.
Newton would certainly agree, that to be fair to all players, all ball weights, all speeds, and all launch angles, FLAT is the only fair situation, and the further we deviate from flat, the more unfair the game becomes.
Lighter bowling balls and slower ball speeds are influenced more in non-flat situations than heavier bowling balls and faster ball speeds.
Further, the gravitational effects of depressions, crowns and tilts have widely varied effects on varied launch angles. The more a bowling lane strays away from flatness, the more those gravitational effects influence different styles of play in different ways.
So now it’s time to continue our testing by redoing each test over and over. The story continues.
Newton…what a guy.